Good morning.
We’ll have a partly sunny Wednesday in Whitewater, with a high of eighty, and southwest winds at 5 to 15 MPH.
I had no idea that Pres. Garfield had an interest in mathematics, but yesterday I learned that he did. While a member of Congress, he produced a simple proof of the Pythagorean theorem, as Esther Inglis-Arkell explains:
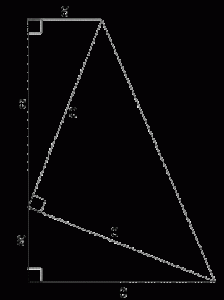
Most will remember the Pythagorean Theorem as the old A2 + B2 = C2 problem, wherein the square of the two sides of a right triangle, added together, will yield the square of the hypotenuse. Garfield’s proof is simple, but it takes a little fiddling with paper.
Grab a piece of paper and cut two identical right triangles, trying to include one long side and one short side. Call the long side A, the short side, B, and the hypotenuse C. Place them, opposing points together, on a piece of paper so that they look like this.
Draw the third line in and you’ve made three triangles. The total area of a triangle can be calculated as one half the base times the height. Each of the original triangles has an area of ½ ab, and the third has an area of ½c2.
A trapezoid has an area calculated by its height times one half the sum of its uneven sides. So the trapezoid has an area of ½ (a+b)(a +b).
Since these areas are the same, this leads us to an equation.
½ ab + ½ ab + ½c2 = ½ (a + b)(a +b)
This simplifies quickly. On the left, the two halves of ‘ab’ can be added together. On the right, we just multiply all the terms.
ab +½c2 = ½ (a2 + b2 + 2ab)
ab +½c2 = ½ a2 + ½ b2 + ab
Since we have ‘ab’ on either side, they cancel each other out.
½c2 = ½ a2 + ½ b2
Multiply it all by 2, and you get:
c2 = a2 + b2
Well, you get that, and the deep satisfaction that you have solved a problem, Presidential-style.
From mathematics to a different sort of puzzle:
Puzzability‘s series this week is about mythology:
Myth Takes
For this week of freaks and Greeks, we started each day with a mythical creature. Then we hid it in a sentence, with spaces added as necessary. The answer spans at least two words in the sentence and starts and ends in the middle of words. The day’s clue gives the sentence with a Greek column in place of the creature.
Example:
I am not rising from this comfortable so
til I’ve finished this fascinating myth about the early days of Mt. Olympus.
Answer:
Faun (sofa until)
What to Submit:
Submit the mythical creature (as “Faun” in the example) for your answer.
Wednesday, August 7:
In Roman mythology, the irides
ora (the goddess Eos in Greek myths) creates a beautiful dawn across the sky.